Probability Theory Basics
Course
888 Learners Already enrolled- Grasp the foundational elements of probability theory and its properties.
- Gain the ability to calculate the probability of complex events using geometrical probability.
- Understand discrete and continuous distributions, such as binomial, Poisson, and Gaussian distributions.
- Explore the concepts of covariance and correlation to measure statistical relationships.
Equip your business with cutting-edge Data and AI expertise.
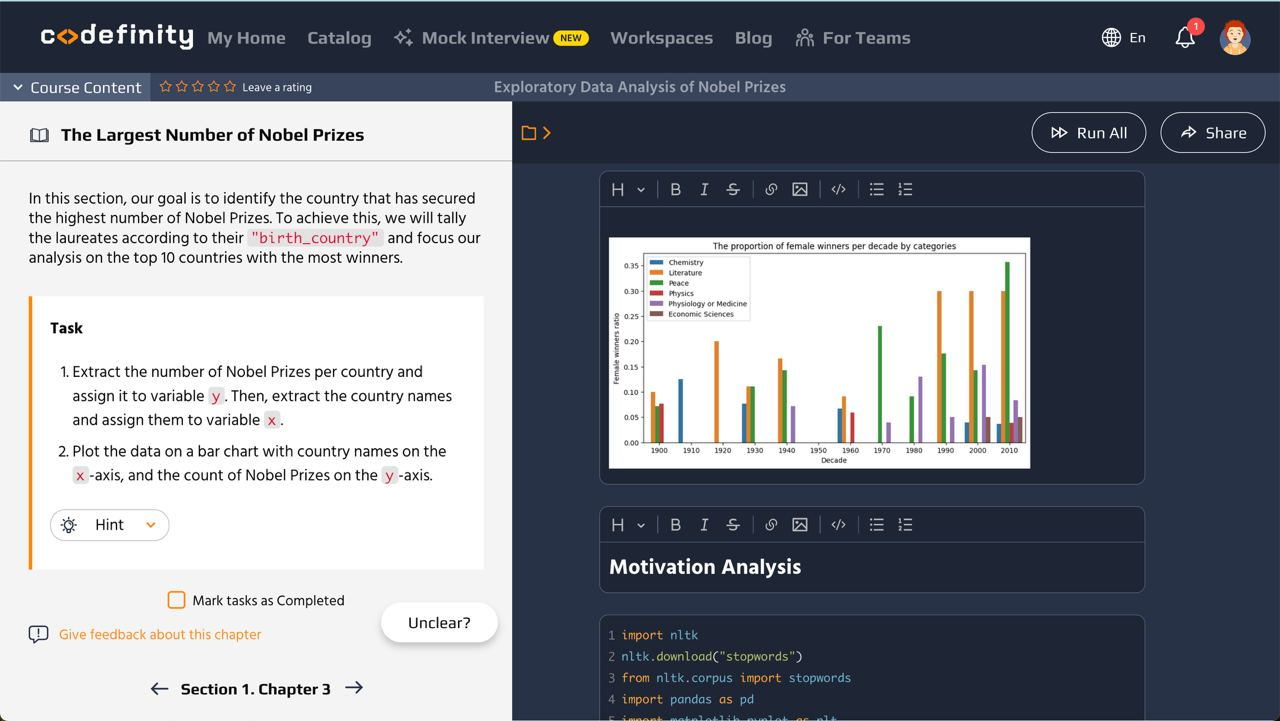
Share it on social media and in your performance review
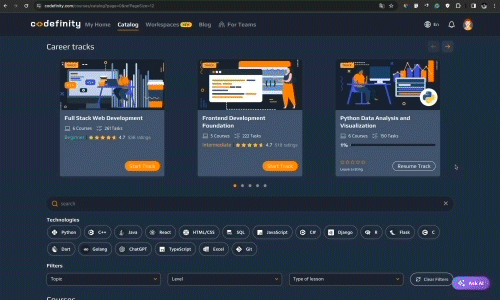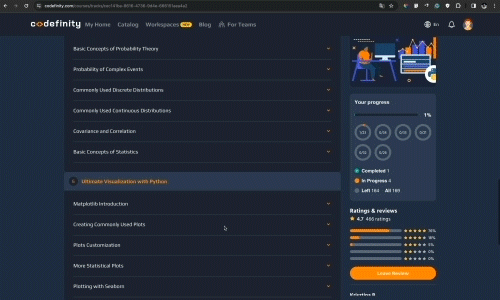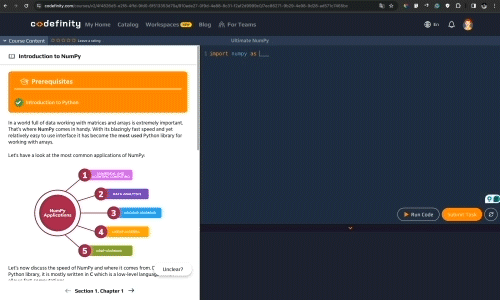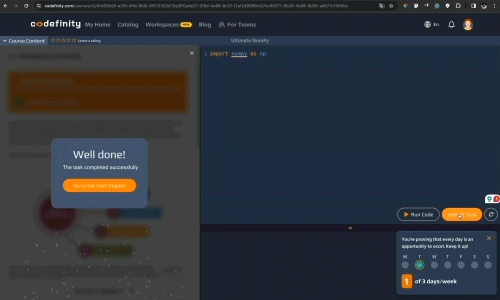
There are 5 modules in this course
Probability theory is a fundamental branch of mathematics that deals with the study of uncertainty and randomness. It provides a framework for understanding and quantifying uncertainty in various fields, including statistics, data analysis, machine learning, finance, physics, and more.Chosen by students of top schools
Why people choose Codefinity for their career
Kwizera Mugisha
The teaching methodology at Codefinity is excellent, and I particularly appreciate how it has prepared me to handle real-world coding problems. Currently, I am delving into Node.js and eagerly anticipate building full-stack projects that integrate all the knowledge I have gained.
Sherry Barnes-Fox
My first course was 4 hours, I did it in a few days, "nugget-style. The instructions are very clear and easy to understand. There is even a hint to help you get the answer, and if you still cannot get the answer, then you can display the answer. I love the learning style that is used, it engages me.
Bill Wagner
I have really liked the browser-based lessons that allow me to code within the lesson. The RUN button allows me to test the code I write before submitting for a grade.
Stephanie Chan
As I went through the first course of the Python track, I liked the way the course was lay out (in easy and digestible modules) with little exercises at the end of each concept.
Daniel Chinea
I have gained a lot of practical and logical thinking skills, along with patience for myself and confidence in myself that I can learn programming.
Steve Bruening
The learning was progressive and made it easy to follow along and make progress. I could feel my skills increasing and building on each other as the course went along.
Learner reviews
4.8
16 reviews
5
81%
4
19%
3
0%
2
0%
1
0%
Showing 3 of 16 reviews
4
Reviewed on Mar 19, 2025
Clear explanation combined with quiz questions that were challenging but not too hard, and the questions were on-topic. Providing AI support also helped a lot.
Andrew graves
5
Reviewed on Dec 5, 2024
good python
.
5
Reviewed on Nov 4, 2024
Recommended if you're interested in learningPython
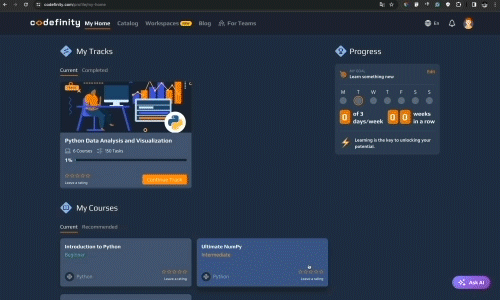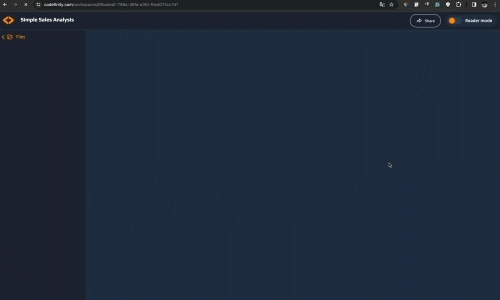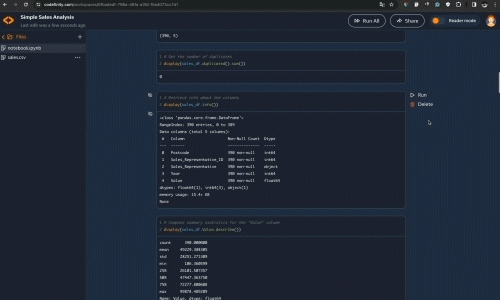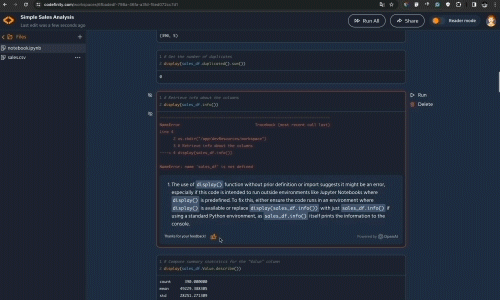
Embrace the fascination of Tech Skills! Our AI-assistant provides real-time feedback, personalized hints, and error explanations, empowering you to learn with confidence.
With Workspaces, you can create and share projects directly on our platform. We've prepared templates for your convenience
Take control of your career development and commence your path into mastering the latest technologies
Real-world projects elevate your portfolio, showcasing practical skills to impress potential employers
Full catalog access
One subscription opens up this course and our entire catalog of projects and skills.
Top-rated, curated contentNew courses added weeklyAI assistant to answer any questionsPassionate community of learners
100+ Top-Rated coursesCompletion certificatesAI-Assistant in all courses
20+ hands-on Real-world projectsPersonalized study tracksUnlimited workspaces100+ Top-Rated coursesCompletion certificatesAI-Assistant in all courses20+ hands-on Real-world projectsPersonalized study tracksUnlimited workspacesYour subscription also includes:
Frequently asked questions
Still have questions?
Write your question here
